Forces on Inclined Planes

example l = 0.4 m, L = 1.2 m, and W = 30 kg, then the weight of W is 30g newtons, so that the force F required to balance the lever is

This force could be produced by suspending a mass of 10 kg at F.

Table of Forces on Inclined Planes

The table below makes it possible to find the force required for moving a body on an inclined plane. The friction on the plane is not taken into account. The column headed “Tension P in Cable per Ton of 2000 Pounds” gives the pull in pounds required for moving one ton along the inclined surface. The fourth column gives the perpendicular or normal pressure. If the coefficient of friction is known, the added pull required to overcome friction is thus easily determined:

Q × coefficient of friction = additional pull required.

Tensions and pressures in pounds.
Per Cent of Grade. Rise, Ft. per 100 Ft.	Angle α	Tension P in Cable per Ton of 2000 Lbs.	Perpendicular Pressure Q on Plane per Ton of 2000 Lbs.	Per Cent of Grade. Rise, Ft. per 100 Ft.	Angle α	Tension P in Cable per Ton of 2000 Lbs.	Perpendicular Pressure Q on Plane per Ton of 2000 Lbs.
1	0.57	20.00	1999.90	51	27.02	908.65	1781.67
2	1.15	39.99	1999.60	52	27.47	922.71	1774.43
3	1.72	59.97	1999.10	53	27.92	936.59	1767.15
4	2.29	79.94	1998.40	54	28.37	950.30	1759.81
5	2.86	99.88	1997.50	55	28.81	963.84	1752.43
6	3.43	119.78	1996.41	56	29.25	977.21	1745.01
7	4.00	139.66	1995.12	57	29.68	990.41	1737.55
8	4.57	159.49	1993.63	58	30.11	1003.44	1730.06
9	5.14	179.28	1991.95	59	30.54	1016.30	1722.54
10	5.71	199.01	1990.07	60	30.96	1028.99	1714.99
11	6.28	218.68	1988.01	61	31.38	1041.52	1707.41
12	6.84	238.29	1985.75	62	31.80	1053.88	1699.81
13	7.41	257.83	1983.31	63	32.21	1066.08	1692.18
14	7.97	277.30	1980.68	64	32.62	1078.11	1684.54
15	8.53	296.68	1977.87	65	33.02	1089.98	1676.89
16	9.09	315.98	1974.88	66	33.42	1101.68	1669.22
17	9.65	335.19	1971.71	67	33.82	1113.23	1661.54
18	10.20	354.31	1968.37	68	34.22	1124.62	1653.85
19	10.76	373.32	1964.85	69	34.61	1135.85	1646.16
20	11.31	392.23	1961.16	70	34.99	1146.92	1638.46
21	11.86	411.03	1957.31	71	35.37	1157.84	1630.77
22	12.41	429.72	1953.29	72	35.75	1168.61	1623.07
23	12.95	448.30	1949.11	73	36.13	1179.22	1615.37
24	13.50	466.75	1944.77	74	36.50	1189.69	1607.68
25	14.04	485.07	1940.29	75	36.87	1200.00	1600.00
26	14.57	503.27	1935.65	76	37.23	1210.17	1592.32
27	15.11	521.33	1930.86	77	37.60	1220.19	1584.66
28	15.64	539.26	1925.93	78	37.95	1230.06	1577.00
29	16.17	557.05	1920.86	79	38.31	1239.80	1569.36
30	16.70	574.70	1915.65	80	38.66	1249.39	1561.74
31	17.22	592.20	1910.31	81	39.01	1258.84	1554.13
32	17.74	609.55	1904.85	82	39.35	1268.16	1546.54
33	18.26	626.76	1899.26	83	39.69	1277.34	1538.96
34	18.78	643.81	1893.55	84	40.03	1286.38	1531.41
35	19.29	660.70	1887.72	85	40.36	1295.30	1523.88
36	19.80	677.44	1881.77	86	40.70	1304.08	1516.37
37	20.30	694.02	1875.72	87	41.02	1312.73	1508.89
38	20.81	710.44	1869.57	88	41.35	1321.26	1501.43
39	21.31	726.69	1863.31	89	41.67	1329.65	1493.99
40	21.80	742.78	1856.95	90	41.99	1337.93	1486.59
41	22.29	758.71	1850.50	91	42.30	1346.08	1479.21
42	22.78	774.47	1843.96	92	42.61	1354.11	1471.86
43	23.27	790.06	1837.34	93	42.92	1362.03	1464.54
44	23.75	805.48	1830.63	94	43.23	1369.82	1457.26
45	24.23	820.73	1823.84	95	43.53	1377.50	1450.00
46	24.70	835.81	1816.98	96	43.83	1385.06	1442.77
47	25.17	850.72	1810.05	97	44.13	1392.52	1435.58
48	25.64	865.46	1803.05	98	44.42	1399.86	1428.43
49	26.10	880.03	1795.98	99	44.71	1407.09	1421.30
50	26.57	894.43	1788.85	100	45.00	1414.21	1414.21

Inclined Plane—Wedge

W = weight of body
Neglecting friction:	If friction is taken into account, then:
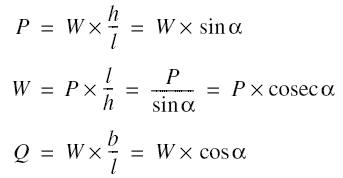	Force P to pull body up is: P=W(μcosα+ sinα) Force P1 to pull body down is: P1 = W(μcosα– sinα) Force P2 to hold body stationary: P2 = W(sinα –μcosα) in which μ is the coefficient of friction.
W = weight of body
Neglecting friction:	With friction:
	Coefficient of friction = μ = tanφ

Neglecting friction:	With friction:
	Coefficient of friction = μ = tanφ P= Wtan(α+φ)

Neglecting friction:	With friction:
	Coefficient of friction = μ. P = 2Q(μcosα+ sinα)

Neglecting friction:	With friction:
	Coefficient of friction = μ = tan φ. P = 2Qtan(α+φ)

Force Moving Body on Horizontal Plane.—F tends to move B along line CD; Q is the component which actually moves B; P is the pressure, due to F, of the body on CD.

Recherche personnalisée